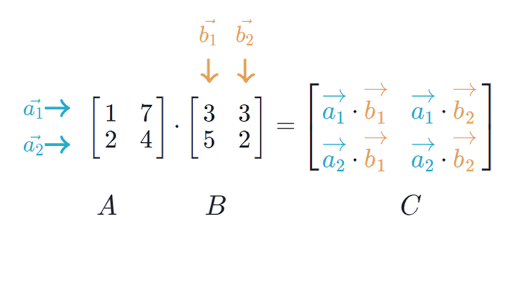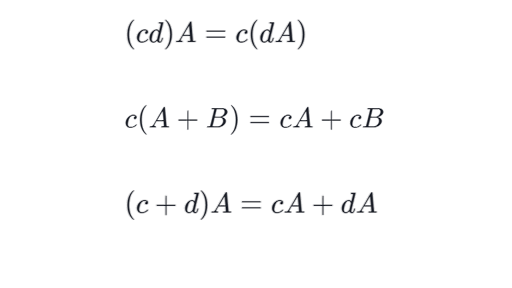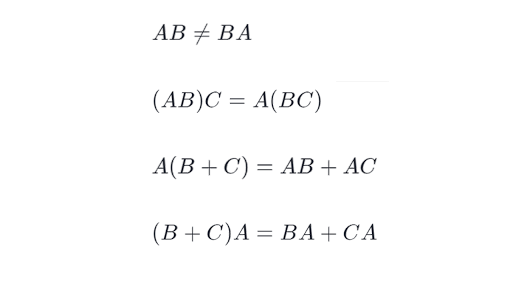Is Vector Matrix Multiplication Commutative
Link of Examples of Matrix Multiplication is given belowhttpsyoutube3ZU812qp9-ELink of Equality of matrices is given belowhttpsyoutubeGbrs1D5l9-Q. Of course if just want to say that it doesnt matter how you write the product itexlambda v v lambdaitex where itexlambdaitex is a scalar and v is a vector then thats trivially true but that is not what commutative means.
Linear Algebra Matrices A First Course In Electrical And Computer Engineering Openstax Cnx
Generally in both of these settings scalar multiplication is only defined on the left.
Is vector matrix multiplication commutative. The scalar scales the vector. Just like for the matrix-vector product the product A B between matrices A and B is defined only if the number of columns in A equals the number of rows in B. In particular matrix multiplication is not commutative.
If p happened to be 1 then B would be an n 1 column vector and wed be back to the matrix-vector. Select the 3D graphics button to see a graphic representation of the rotations. The one exception occurs when the two rotations occur in the same plane.
The addition of vectors is commutative because. Finding the values of a vector if the vectormatrix product and the value of the matrix is known only using left multiplication operations 6 Commutative property of matrix multiplication or lack thereof. Multiplication of a vector by a scalar changes the magnitude of the vector but leaves its direction unchanged.
A b b a displaystyle vec a vec b vec b vec a. In math terms we say we can multiply an m n matrix A by an n p matrix B. Two well-known examples of commutative binary operations.
The scalar changes the size of the vector. Even if m q then A B is of order m q but B A is of order p q. Notably absent is commutativity.
Where satisfies a few properties. If A is of order m n and B is of order p q then A B is defined if n p but B A is not defined unless m q. AB AC does not imply B C even when A B 0.
AB is not usually equal to BA even when both products are defined and have the same size. The matrices button shows. In such cases the commutative law for multiplication is valid.
That is for matrices A and B A B B A in general. Matrix Multiplication is not commutative in general. Matrix multiplication is not commutative.
Further when they form an algebra over when granted the usual matrix multiplication. We know that scalars are equal to their own transpose so mathematically we have. The addition of real numbers is commutative since.
You cannot switch the order of the factors and expect to end up with the same result. One way to see why the vector multiplication is commutative is to notice that the result of bsx textTy is a scalar. Matrix multiplication does not satisfy the cancellation law.
Scalar multiplication is the product of a scalar and a vector- you cant interchange them. That is for a vector spacealgebra over the field we define.
Is Matrix Multiplication Commutative Video Khan Academy
Pin On Colleges
Multiplying Matrices Article Matrices Khan Academy
Linear Algebra Fundamentals For Machine Learning Michael W Brady
Matrix Multiplication E2
Pin On Mathematics
Pin Auf Dp Ai
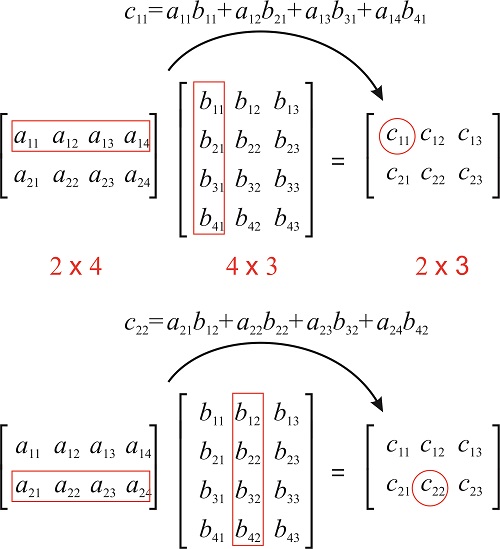
15 3 Matrix Multiplication Chemistry Libretexts
Properties Of Matrix Scalar Multiplication Article Khan Academy
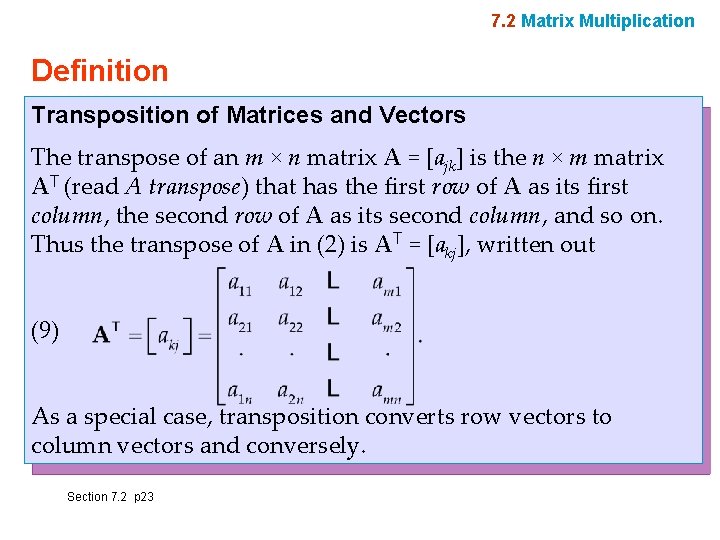
7 1 Matrices Vectors Addition And Scalar Multiplication
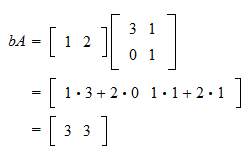
Matrix Multiplication And Linear Combinations
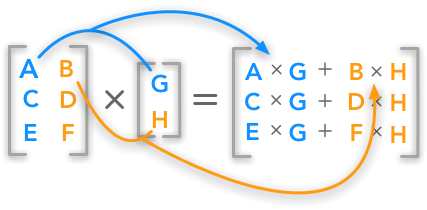
Introduction To Matrices And Vectors Multiplication Using Python Numpy
Pin On 10 Math Problems
Matrix Multiplication An Overview Sciencedirect Topics
What Does Span Mean In Linear Algebra Algebra Linear Math
An Intuitive Guide To Linear Algebra Algebra Matrix Multiplication Linear
Pin On Bob S Stuff
Properties Of Matrix Multiplication Article Khan Academy
Pin On High School Math
